1000

Asia Pacific J Clin Nutr (1996) 5(4): 217-221

Multi-frequency bioelectrical impedance for the prediction of body water compartments: validation in different ethnic groups

Paul Deurenberg¹ PhD, Anna Tagliabue² MSc, Jingzhong Wang³ BSc, Zewdie Wolde-Gebriel⁴ PhD

1. Department of Human Nutrition, Wageningen Agricultural University, The Netherlands;
2. Department of Human Nutrition, University of Pavia, Italy;
3. Institute of Nutrition and Food Hygiene, Chinese Academy of Preventive Medicine, Beijing, China;
4. National Research Institute on Health and Nutrition, Addis Ababa, Ethiopia

Plenary lecture presented at an APCNS Satellite Meeting of the Asian Congress of Nutrition on "Nutrition, Body Composition and Ethnicity" in Tianjin, China on 5th October 1995.

---

Body weight, body height and impedance at 1 kHz and at 100 kHz were measured in the fasting state in groups of healthy adult males and females from Ethiopia, China, Italy and The Netherlands. Total body water (TBW) and extracellular water (ECW) were determined by deuterium oxide and bromide dilution respectively. TBW/height and ECW/height were calculated as a measure of body build.

The relation between TBW and ECW as measured by dilution technique and impedance index (height²/impedance) at 100 kHz and 1 kHz respectively was not different between the four populations. When a prediction formula for TBW and ECW from impedance index, developed in another (Dutch) population was applied to the four country groups, TBW was slightly overestimated, varying from 0.1 ± 1.8kg in the Italian group to 0.6 ± 1.8kg in the Dutch group. Also ECW was slightly but significantly overestimated, varying from 0.3 ± 0.9kg in the Dutch group to 1.1 ± 0.9kg in the Italian group.

The bias for TBW was correlated with TBW/height in all country groups (correlation coefficients ranging from 0.33 to 0.56, all p<0.05) and the bias for ECW was correlated with ECW/height in all country groups (correlation coefficients ranging from 0.52 to 0.63, all p<0.05) except in the Ethiopians (r=0.23, p<0.1). Moreover, the bias for TBW and ECW was, in two of the four country groups, correlated with body water distribution (ECW/TBW). When the differences between measur 1000 ed and predicted TBW and ECW were corrected for differences in TBW/height and ECW/height and for differences in body water distribution, the bias significantly decreased and was not different from zero any more.

It is concluded that multi-frequency bioelectrical impedance is an appropriate technique to predict body water compartments in populations. Difference between (ethnic) groups can be partly attributed to differences in body build.

Key words: bio-electrical impedance, dilution techniques, total body water, extracellular water, body composition, body build, validation, ethnicity

---

Introduction

Body composition is an important parameter for the determination of the nutritional status¹. Of the different aspects of body composition that can be measured, total body water (TBW) and extracellular water (ECW) are of special importance. In many diseases, as in edema or in dehydration, the distribution of body water over the extra and intra cellular space is disturbed. For the determination of TBW and ECW dilution methods can be used^1,2. They have, however, the limitation that they are rather laborious and time consuming and need skilled personnel. Bioelectrical impedance can be used to assess body water³. When body impedance is measured at low (1-5kHz) and high frequency (50-100kHz) the amount of extra-respectively total body water can be assessed using empirically derived prediction formulas^4-6. Generally prediction formulas have the limitation that they are population specific⁷. As total body impedance is strongly determined by impedance of the arm and of the leg^8,9, which in turn in determined by the length and the cross-sectional area of the extremities, differences in body build will have an impact on the validity of the predicted value. Also body water distribution has an impact on the validity of predicted values^6,7,10.

Existing prediction formulas have been developed in Western, mostly Caucasian populations. Different ethnic groups may have a different body build and body water distribution, which would limit the use of Caucasian prediction formulas in these populations. Some studies indicate differences in the relationship between body composition and body impedance in different ethnic groups^11-13.

The aim of this study was to test the validity of prediction formulas for ECW and TBW from bioelectrical impedance in different (ethnic) groups and to relate the bias of predicted values to body water distribution and measures of body build.

Subjects and methods.

In total, 172 healthy subjects, 89 males and 83 females participated in the study. They were recruited in four countries (cities), Ethiopia (Addis Ababa), China (Beijing), Italy (Pavia) and The Netherlands (Wageningen). The subjects cannot be regarded as representative for their country, however they were not specially selected. The aim of the study was explained to the subjects. The study protocol was in accord with the guidelines of the Declaration of Helsinki of the World Medical Association (1989) and was approved by the Medical Ethical Committee of the Department of Human Nutrition, Wageningen, The Netherlands. In each centre the same study protocol was used.

All measurements were performed in the morning, in the fasting state after emptying the bladder. Body weight in underwear or swimsuit was measured to the nearest 0.1 or 0.5kg (Ethiopia) and body height without shoes to the nearest 0.1 or 0.5cm (Ethiopia). Body mass index was calculated as weight/height squared (W/H², kg/m²). After that, 1000 body impedances at 1 and 100kHz were measured on the left side of the body immediately after lying supine using a HUMANIM SCAN (Dietosystem, Milan, Italy) multi-frequency impedance analyser. The self-adhesive electrodes (Littman 3M, 2325 VP, St. Paul MN, USA) with a surface area of about 5cm² were attached as described by Lukaski et al¹⁴. From impedance, the impedance index was calculated as height squared/impedance at frequency f (H²/Z_f, cm²/W). From impedance index at 1kHz and at 100kHz, extracellular water (ECW) and total body water (TBW) were predicted with the respectively formulas⁶:

ECW (kg) = 0.24253*H²/Z₁ + 4.1 and

TBW (kg) = 0.51303*H²/Z₁₀₀ + 6.3

In addition, ECW and TBW were calculated with prediction formulas that included not only impedance index but also weight and age, and, for TBW, also sex⁶.

Total body water and extracellular water were determined by dilution techniques. A cocktail of an accurately weighed dose of about 15g deuterium oxide and 900mg bromide (1.34g as potassium bromide) was taken orally by the subjects. After 2.5 to 3 hours dilution time a venous blood sample was taken, plasma was separated and stored at -80° C until analysis. Deuterium in plasma was determined after sublimation by infrared spectroscopy¹⁵. TBW was calculated using a correction factor of 0.95 for non aqueous dilution¹. Bromide in plasma was determined by HPLC after ultra filtration¹⁶. ECW was calculated using a correction factor of 0.9 for non-extracellular distribution¹ and a correction factor of 0.95 for the DONNAN effect¹. All analyses were done in the same laboratory (Wageningen).

1000

As a crude measure of body build, TBW/height and ECW/height were calculated. A slender subject will have lower values of these parameters compared to a more plump subject. Body water distribution and measures of body build in the country groups were compared with the values of these parameters of the population in which the prediction formulas were developed (reference population). The bias of predicted values of TBW and ECW was corrected for differences in body build and body water distribution compared to the reference population. The SPSS program17 was used for statistical analysis. Differences between measured and predicted values (bias) were tested for significance with the paired t-test. Correlations are Pearson’s product moment correlations. Differences in variables between groups (countries) were tested with ANOVA or ANCOVA (analysis of co-variance). Multiple regression analyses were performed using country as a dummy variable. Regression equations were tested for differences in slope and/or intercept with the technique described by Kleinbaum and Kupper18. The validity of predicted values is described according to Bland and Altman19. Values are expressed as mean ± standard deviation (SD) except for regression coefficients for which the standard error of the mean (SE) is shown

Table 1. Characteristics of the study groups. 1000 1000   Ethiopia China Italy Netherlands   mean SD mean SD mean SD mean SD Males 24   22   20   23   Females 20   23   20   20   Age (years) 34.2 6.3 31.5 6.4 22.0 2.0 31.4 4.5 Weight (kg) 56.4 10.1 58.3 10.2 64.6 11.1 74.4 13.5 Height (cm) 163 9 165 7 170 10 176 10 BMI (kg/m2) 21.3 3.4 21.3 2.6 22.2 2.5 24.1 4.8 TBW (kg) 26.9 5.2 34.7 5.9 35.6 7.4 39.7 7.0 ECW (kg) 11.7 1.6 13.9 2.3 14.1 2.5 16.2 2.4 Abbreviations: BMI = body mass index; TBW = total body water; ECW = extracellular water

Results Table 1 provides characteristics of the subjects of the four countries. The sex distribution was comparable between the countries. Most pronounced differences between the groups were the lower age of the Italians, the low values of body water compartments in the Ethiopians and the high body weight, body height and body mass index of the Dutch subjects. In Table 2 the coefficients of the regression equations between TBW and H2/Z100 and ECW and H2/Z1 are given for the four groups. The four regression equations for TBW and the four regression equations for ECW did not differ in slope and intercept. 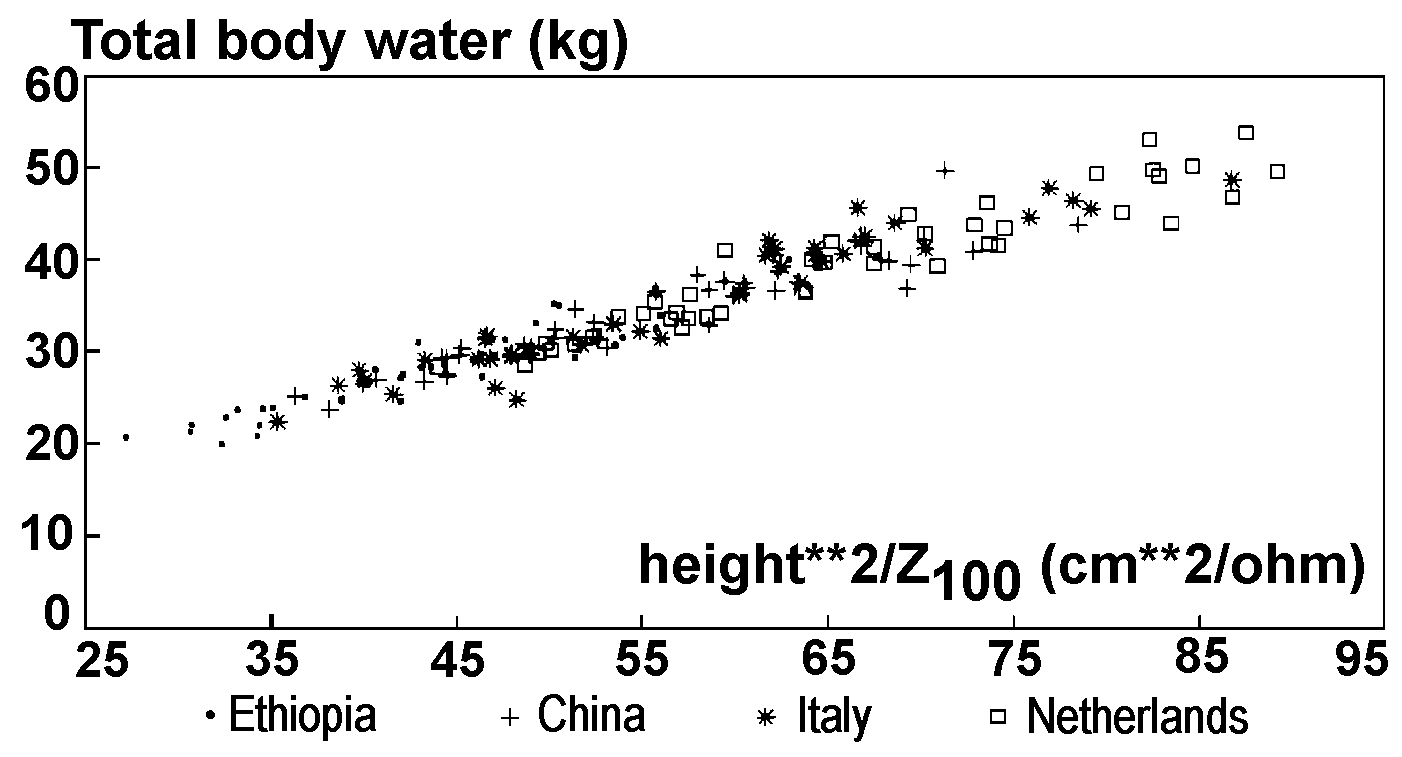Figure 1 and Figure 2 show the relationship between TBW and H2/Z100 and ECW and H2/Z1 respectively for all subjects combined.

Table 2. Regression coefficients for total body water and extra cellular water against impedance index.   Total body water Extracellular water   H2/Z100 intercept H2/Z1 intercept   mean SE mean SE mean SE mean SE Ethiopia 0.523 0.010 5.6 0.6 0.258 0.007 3.1 0.4 China 0.526 0.011 6.0 0.7 0.253 0.009 3.4 0.5 Italy 0.522 0.011 5.7 0.8 0.255 0.008 3.3 0.4 Netherlands 0.526 0.011 5.9 0.8 0.244 0.008 4.0 0.4 Abbreviations: H = body height; Z100 = impedance at 100 kHz, Z1 = impedance at 1 kHz

Figure 1. Relation between total body water and impedance index at 100kHz.
Figure 2. Relation between extracellular water and impedance index at 1kHz.	1000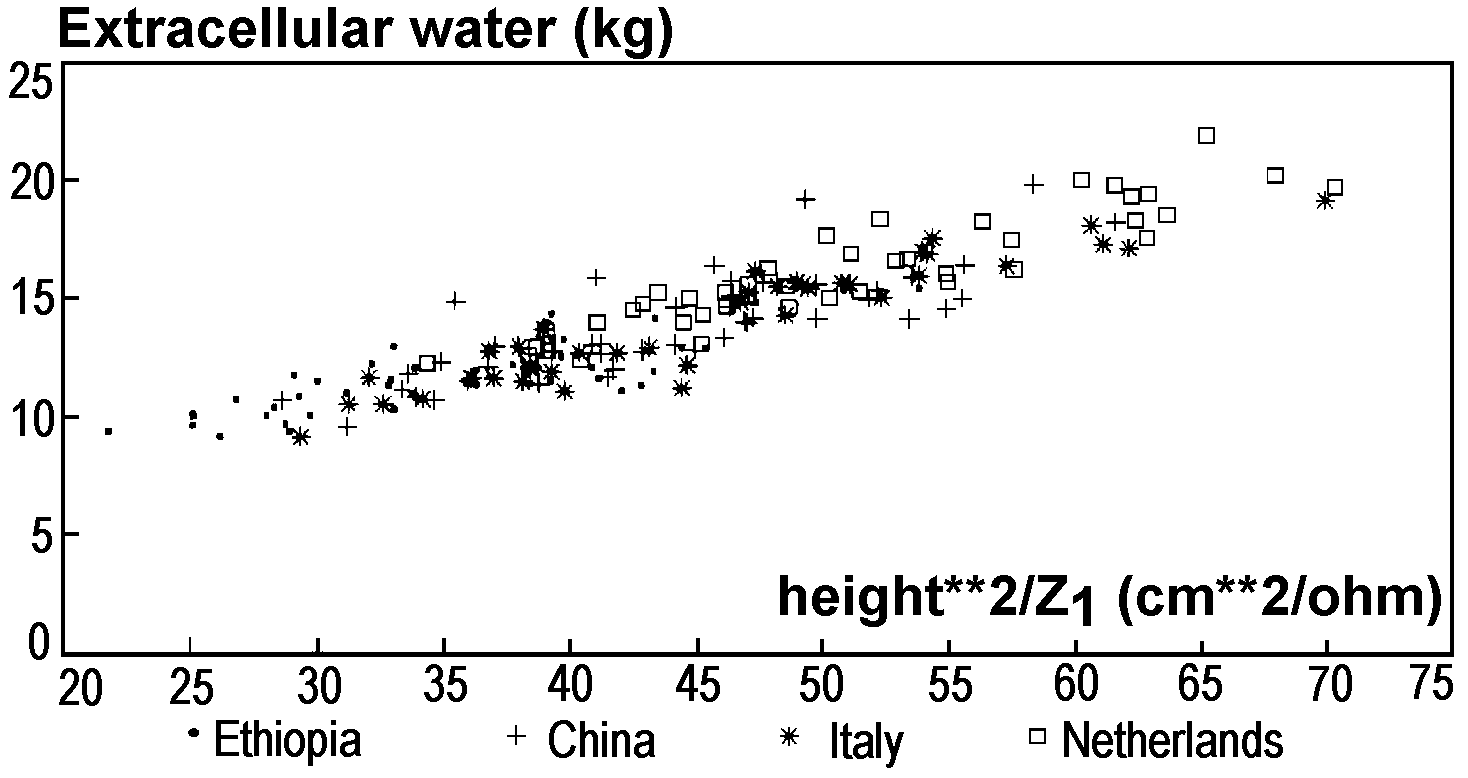

In Table 3 the differences between measured and predicted TBW and ECW (bias) from impedance index alone, using prediction equations from the literature, are given. Although the bias for ECW was significantly different from zero in all groups it was relatively small and did not differ between the countries. The bias of ECW and TBW correlated with body water distribution (ECW/TBW) and with body build (TBW/height and ECW/height). The correlation coefficients are given in Table 4 for the separate countries. As can be read from the table both TBW and ECW were slightly over predicted at higher levels of the body water compartment. The bias was correlated with body water distribution in most country groups and with measures of body build in all country groups. The positive correlation of the bias with body build means that in slender subjects, thus in subjects with a relatively low value of TBW/height or ECW/height, the prediction formulas tend to overestimate total body water and extracellular water.

Table 3. Differences between measured and predicted total body water and extracellular water.   Ethiopia China Italy Netherlands   mean SD mean SD mean SD mean SD d TBW (kg) -0.5 1.7 1000 -0.3 1.8 -0.1 1.8 -0.6* 1.8 d ECW (kg) -1.0* 1.0 -0.9* 1.1 -1.1* 0.9 -0.3* 0.9 * p<0.05 from zero; Abbreviations: d TBW = measured minus predicted total body water; d ECW = measured minus predicted extracellular water

Table 4. Correlation of the bias of predicted total body water and extracellular water with body water distribution and measures of body build. 1000   Ethiopia China Italy Netherlands d TBW with:         mean TBW 0.25 0.19 0.40* 0.27 ECW/TBW -0.22 0.09 -0.51* -0.38* TBW/H 0.38* 0.33* 0.56* 0.47* d ECW with:         mean ECW -0.18 0.29 0.28 0.26 ECW/TBW 0.62* 0.73* 0.23 0.18 ECW/H 0.23 0.63* 0.52* 0.58* * p<0.05 Abbreviations: TBW = total body water; mean TBW = mean of measured and predicted TBW; d TBW = measured minus predicted TBW; ECW = extracellular water; mean ECW = mean of measured and predicted ECW; d ECW = measured minus predicted ECW; H = body height;

Table 5. Body water distribution and body build in the four studied groups and in the reference group.   Ethiopia China Italy Netherlands Referencea   mean SD mean SD mean SD mean SD mean SD ECW/ TBW 0.41 0.03 0.40* 0.03 0.40* 1000 0.02 0.41 0.02 0.42 0.03 TBW/H (kg/cm) 0.18* 0.03 0.21* 0.03 0.21* 0.03 0.22 0.03 0.22 0.03 ECW/H (kg/cm) 0.07* 0.01 0.08* 0.01 0.08* 0.01 0.09 0.01 0.09 0.01 a) reference: Dutch group in which the prediction formulas were developed; * p<0.05 compared to reference population

< 1000 tr>

Table 6. Mean bias of total body water and extracellular water corrected for body water distribution and body builda. 1000 1000   Ethiopia China Italy Netherlands   < 1000 font size="1">mean SD mean SD mean SD mean SD d TBW corrected for:           - -0.5* 1.7 -0.3 1.8 -0.1 1.8 -0.6* 1.8 ECW/TBW -0.5* 1.7 -0.3 1.8 -0.3 1.8 -0.7* 1.7 TBW/H 0.0 1.6 -0.1 1.7 0.1 1.7 -0.6* 1.8 ECW/TBW 0.0 1.6 0.0 1.7 0.0 1.7 -0.5 1.7 and TBW/H                 d ECW corrected for:           - -1.0* 1.0 -0.9* 1.1 -1.1* 0.9 -0.3 0.9 ECW/TBW -0.9* 0.8 -0.6* 0.9 -0.9* 0.9 -0.2 0.9 ECW/H -0.4 0.9 -0.6* 0.9 -0.8* 0.9 -0.3 0.9 ECW/TBW -0.2 0.7 -0.4 0.8 -0.5* 0.7 -0.2 0.7 1000 and ECW/H                 a) corrected for differences in indicated parameters compared to the reference group in which the prediction formulas were developed *p<0.05

Table 5 shows body water distribution and body build in the four groups in comparison with the population in which the prediction formulas were developed (reference population). In some population groups, body water distribution and body build were different compared to the reference group. These differences were apparent in both males and females (data not shown). Table 6 shows the bias of TBW and ECW in each group corrected for differences in body water distribution and/or measures of body build compared to the reference population. In most groups the mean bias in predicted values decreased, sometimes markedly, after correction for these parameters. Also the individual error, which is indicated by the SD of the bias, lowered.

When prediction formulas not only containing the impedance index but also weight, age and sex⁶ were applied to the subjects, the bias of ECW was -0.8 ± 1.0kg, -0.4 ± 1.1kg, -1.2 ± 0.8kg and -0.6 ± 0.7kg and for TBW -0.7 ± 1.5kg, 1.0 ± 1.8kg, -0.9 ± 1.5kg and -0.6 ± 1.5kg for Ethiopian, Chinese, Italian and Dutch subjects respectively. The bias of ECW showed comparable correlations with body water distribution as in Table 4, but was only in the Chinese group correlated with ECW/height. The bias of TBW was not correlated with body water distribution, nor with TBW/height in either group.

Discussion

In this study, prediction formulas for TBW and ECW developed in a Caucasian population were validated in several independently measured groups, partly with a different ethnic background. These groups cannot be regarded as representative for the entire population of that country, but they were not specially selected. The study design and the methodology used in this study was equal for all groups and the chemical analysis to obtain reference values for ECW and TBW were performed in the same laboratory. The impedance instruments used were from the same manufacturer. It is known from the literature^20,21 that different impedance instruments can give different readings, even when the same subject is measured. In three out of four groups (Ethiopia, China, The Netherlands) impedance was measured by the same investigator. Thus it is likely that differences found between groups are not due to differences in methodology and/or standardisation.

The inclusion of other independent variables than impedance index in a prediction formula generally lowers the prediction error. However, it also makes a prediction equation more population specific⁷. Therefore the main statistical analyses were performed using prediction equations containing only the impedance index as independent variable. The results show (Table 3) that the mean bias, in all four groups, was relatively small and comparable, for TBW as well as for ECW. This was to be expected as the regression equations for TBW and ECW did not differ between the groups (Table 2). The bias for TBW and ECW was not correlated with the mean value of predicted and measured ECW and TBW respectively, except for the Italian subjects for TBW. This is an important condition for a valid prediction¹⁹. However, generally the bias of ECW was positively correlated with body water distribution and the bias of TBW was negatively correlated with body water distribution. This was found by us in earlier studies^6,22,23. For ECW it can be explained that, at low frequency, the current is partly conducted along the cell membrane²⁴, resulting in biased (lower) impedance values and hence causing an over prediction of ECW. For TBW it is likely that body impedance is influenced by the different specific resistivities of intra and extracellular fluid^6,10. The phenomenon is discussed in detail elsewhere²³. The bias of predicted TBW and ECW was also correlated with measures of body build. From a theoretical point of view, slender subjects have higher impedance values compared to subjects with a plumper body build⁷. As most of the water is located in the trunk which only shows a minor contribution to total body impedance^8,9, plump subjects will have relatively high impedance values compared to their amount of water. Hence, prediction formulas developed in plump subjects will overestimate body water in more slender subjects. This is confirmed by the positive correlation between bias and TBW/height or ECW/height.

After correction for differences in body water distribution (ECW/TBW) and/or for differences in body build (TBW/height and ECW/height) between the groups under study and the group in which the prediction formulas were developed, the bias decreased and was not statistically significant any more. This clearly confirms the dependency of body impedance on body build and shows that ideally population specific prediction formulas have to be used. The fact that, also in the Dutch group, the validity of the prediction was affected by body water distribution and body build, shows that even in a very comparable group these effects have an impact. The comparison in the four different groups confirms an earlier statistical analysis of the Italian and Dutch groups²⁵ where the differences in the relationship between body water compartments and impedance were studied in relation to a number of anthropometric variables.

When prediction formulas for TBW and EC 1000 W were used that also contained body weight, age and (for TBW) sex, the bias in predicted ECW generally decreased, but the bias for TBW slightly increased. With these prediction formulas, however, there was no effect of body build any more. It seems likely that including other parameters in the regression equation, especially weight, the effect of body build is taken into account.

Summary

Prediction formulas for TBW and ECW, developed in a Dutch Caucasian population, showed generally valid values in Ethiopian, Chinese and Italian subjects as well as in another group of Dutch subjects. The small bias was generally dependent on body water distribution and measures of body build, and decreased after correction for differences in body water distribution and body build.

Acknowledgments

We would like to thank the participants for volunteering in the study, Mr Frans JM Schouten for the chemical analyses of the blood samples and Mrs Hellas Cena, Mr Asfaw Tesema and Mrs Xiaogui Wang for their help in the conduct of the study. The study was funded in part by Dietosystems (Milan, Italy).

---

Multi-frequency bioelectrical impedance for the prediction of body water compartments: validation in different ethnic groups

Paul Deurenberg, Anna Tagliabue, Jingzhong Wang, Zewdie Wolde-Gebriel

Asia Pacific Journal of Clinical Nutrition (1996) Volume 5, Number 4: 217-221

---

References

1. Forbes GB. Human Body Composition. New York: Springer Verlag, 1987.
2. Deurenberg P. The assessment of body composition: use and misuse. Annual Report Nestle Foundation 1992, 35-72.
3. Hoffer EC, Meador CK, Simpson DC. Correlation of whole body impedance with total body water J Appl Physiol 1969; 27: 531-534.
4. Segal KR, Burastero S, Chun A, Coronel P, Pierson RN, Wang J. Estimation of extra-cellular water and total body water by multiple frequency bioelectrical-impedance measurements. Am J Clin Nutr 1991; 54: 26-29.
5. Van Marken Lichtenbelt WD, Westerterp KR, Wouters L, Luijtendijk SCM. Validation of bioelectrical impedance measurements as a method to estimate body water compartments. Am J Clin Nutr 1994; 60: 159-166.
6. Deurenberg P, Tagliabue A, Schouten FJM. Multi-frequency impedance for the prediction of extra cellular water and total body water. Brit J Nutr 1995;73:349-358
7. Bioelectrical impedance analysis in body composition measurement. NIH Technol Assess Statement 1994 December 12-14;1-35
8. Baumgartner RN, Chumlea WC, Roche AF. Estimation of body composition from bio-electrical impedance of body segments. Am J Clin Nutr 1989;50:221-226
9. Fuller NJ, Elia M. Potential use of bioelectrical impedance of the whole body and of body segments for the assessment of body composition: comparison with densitometry and anthropometry. Eur J Clin Nutr 1989;43:779-792
10. Deurenberg P, van der Kooy K, Leenen R, Schouten FJM. Body impedance is largely dependent on the intra and extracellular water distribution. Eur J Clin Nutr 1989;43:845-53
11. Zillikens MC, Conway JM. The estimation of total body water by bioelectrical impedance analysis in blacks. Am J Hum Biol 1991; 3: 25-32
12. Heymsfield SB, Wang Z-M. Bio-impedance analysis: modelling approach at the five-levels of body composition and influence of ethnicity. Age & Nutrition 1994;5:108-110
13. Wang J, Thornton JC, Burastero S, Heymsfield SB, Pierson RN. Bio-impedance analysis for estimation of total body potassium, total body water and fat free mass in white, black and Asian adults. Am J Hum Biol 1995;7:33-41
14. Lukaski HC, Johnson PE, Bolonchuck WW, Lykken GE. Assessment of fat free mass using bioelectrical impedance measurements of the human body. Am J Clin Nutr 1985;41:810-817
15. Lukaski HC, Johnson PE. A simple, inexpensive method of determining total body water using a tracer dose of D20 and infrared absorption of biological fluids. Am J Clin Nutr 1985;41:363-370
16. Miller ME, Cappon CJ. Anion exchange chromatographic determination of bromide in serum. Clin Chem 1984;30:781-783
17. SPSS/PC. V4.0 Manuals. Chicago, IL.: SPSS Inc, 1990.
18. Kleinbaum DG, Kupper LL. Applied regression analysis and other multivariable methods. North Scituate, Massachusetts, Duxbury Press, 1978.
19. Bland JM, Altman DG. Statistical methods for assessing agreement between two methods of clinical measurements. Lancet 1986;I:307-310
20. Deurenberg P, van der Kooy K, Leenen R. Differences in body impedance when measured with different instruments. Eur J Clin Nutr 1989;43:885-886
21. Heitmann BL. Evaluation of body fat estimated from body mass index, skinfolds and impedance: a comparative study. Eur J Clin Nutr 1990;44:831-837
22. Visser M, Deurenberg P, van Staveren WA. Multi-frequency bio-electrical impedance for assessing total body water and extra-cellular water in the elderly. Eur J Clin Nutr 1995;49:256-266
23. Deurenberg P. Multi-frequency impedance as a measure of body water compartments. In: Body composition techniques and assessment in health and disease (PSW Davies & TJ Cole, eds. 45-57). Cambridge University Press, 1995
24. Rush SR, Abildskov JA, McFee S. Resistivity of body tissues at low frequencies. Circ Res 1963;12:40-50
25. Tagliabue A, Cena H, Deurenberg P. Comparative study of the rela-tionship between multifrequency impedance and body water compart-ments in two European populations. Brit J Nutr, 1996; 75:11-19.

Copyright © 1996 [Asia Pacific Journal of Clinical Nutrition]. All rights reserved.
Revised: January 19, 1999 .

to the top

0