1000

Asia Pacific J Clin Nutr (1995) 4: 177-179

A new equation set for converting body density to percent body fat

R. H. Nord and R. K. Payne

Norland Corporation, Fort Atkinson, Wisconsin, USA.

One source of error in the underwater weighing (UWW) method of body fat determination is variability in bone mass as a fraction of total nonfat mass. We examined this error theoretically and experimentally using data gathered on 219 human subjects who were measured by UWW and also by the newer technique of dualenergy X-ray absorptiometry (DXA). The experimental data suggest an error was made in the assumed bone mass fraction used in the derivation of the Brozek equation, the standard means of converting the body density values obtained in UWW to body fat percent. Using this new experimental data, a new equation (set) is proposed for use in UWW measurements.

Introduction

Underwater Weighing (UWW) has been an accepted method of determining the fat content of the human body for many years. Dual-energy X-ray absorptiometry (DXA) is a relatively new method for determining the bone mineral content and also the fat content of the body. One of the weaknesses of UWW is its sensitivity to variability of bone mass as a fraction of the lean compartment in different individuals. Since DXA differentiates bone mineral and measures it independently, it is possible to use DXA data to investigate the error in UWW. In doing so we found two ways in which the UWW method could be improved. We developed a new equation set for calculation of body fat percent from body density which (a) takes into account a difference in bone mass fraction between the sexes, and (b) corrects an error in the average value for bone mass fraction which was used in the derivation of the widely used Brozek equation.

Theoretical sensitivity of UWW to bone mass

The standard equations used in UWW assume that bone mineral, which has a relatively high density, will be a fixed fraction of the lean compartment. Any deviation from this fraction in an individual will result in an error in calculated percent fat. Figure I shows this error computed theoretically for typical subjects using the tissue densities and the bone mineral fraction of 4.8% given by Brozek¹. Note that 0.048 is the value at which the %FAT error is zero. Since DXA is able to measure bone mineral mass independently, there is no similar error in DXA.

Figure 1. Theoretical error in percent fat as a function of bone mass fraction of total nonfat mass (typical subject 55 kg).

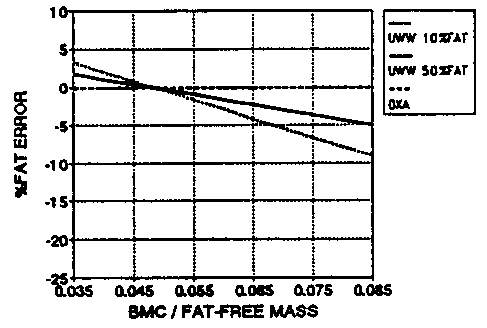
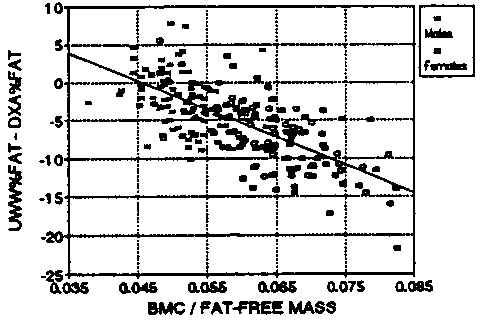
Figure 2. Experimental difference between UWW and DXA percent fat measurements as a function of bone mass fraction as measured by DXA. Mean values for BMC/FFM are 0.052 for men and 0.065 for women. Data from St Luke's Hospital² and the Un 1000 iversity of Wisconsin³.

Experimental results

In the process of calibrating Norland's DXA body composition software, both DXA and UWW measurements were made on 219 volunteer subjects. The measurements were made at two sites, the Body Composition Unit at St Luke's Hospital in New York City², and the Department of Sports Medicine at the University of Wisconsin in Madison³.

The DXA measurement provides a measure of the confounding factor (bone mass) and also a measure of body fat percent which is expected to be free of this error. The parameter which is related to the error is the ratio of bone mineral mass (BMC) total fat-free mass (FFM). The difference between percent fat by UWW and by DXA is plotted versus the ratio BMC FFM in Fig.2. Compare this plot with the theoretical plot of Fig. l .

The experimental data follow approximately the theoretical linear distribution, and the regression line crosses the zero error axis at 0.044, which is close to value of 0.048 used in the development of the Brozek equation. The fact that the slope of the regression line is greater than expected may be an indication of yet another dependency on bone mass in one of the techniques, although at present we do not know what it is.

What is clear from the data is that the mean value of BMC FFM for this population is not 4.8% as given by Brozek, and that there is a significant difference in this parameter for men and women. The mean values we obtained are 5.2% for men, and 6.5% for women.

An improved underwater weighing equation

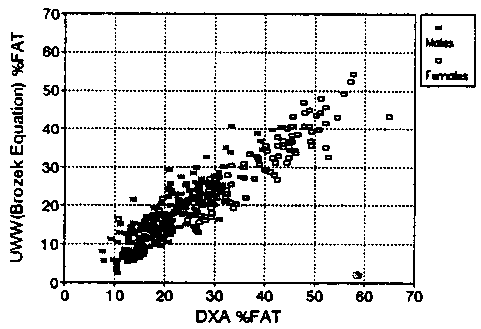
Figure 3 compares the percent fat (%FAT) values from UWW with those of DXA on 219 human subjects. The standard Brozek equation was used in the UWW calculations. Note that the agreement is not very good, with a regression slope of 0.84.

Figure 3. Percent body fat by UWW and by DXA compared; standard Brozek equation used to calculate UWW result. Regression: UWW%FAT = 0.843 * DXA%FAT - 1.14. Data from St Luke's Hospital² and the University of Wisconsin³.

In a previous paper⁴, from examination of the same experimental data, we suggested that there may be a systematic error in the Brozek equation and that this may be the source of the disagreement between the two methods.

We therefore decided to develop a new version of the Brozek equation (actually a set of two because of the sex difference). In addition to using different values for BMC/FFM as set out above, we used a slightly different value of the density of lean soft tissue, which we calculated from published body data, as follows:

Woodard and White⁵ give typical values of the composition of 36 tissue groups or organs, as percent water, lipid, protein, ash, and other materials such as carbohydrates. White, Woodard, and Hammond⁶ give the masses of nearly the same list of tissue groups and organs for a typical male and female. We combined these data to give the total masses of the water, protein plus carbohydrates, and ash in non-skeletal parts of the typical male and female bodies. The resulting mass fractions were used, with densities for the component materials given by Brozek¹, to obtain a combined density for the lean soft tissue compartment. Our value for lean tissue density is 1.0736, compared with Brozek's value of 1.0694.

Derivation f19 of the new equations

Derivation of the new equations consists of combining densities according to the rule

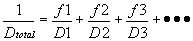
where D_total is the combined density of a combination of materials having densities Dl, D2, D3, . . ., according to the proportion given by mass fractions fl, f2, f3, ....

We used the following parameters:

D_bone = 2.98 (Brozek)

D_fat (lipid) = 0.915 (Brozek)

D_lean (non-bone) = 1.0736 (prev. section)

BMC FFM (Men) = 0.0523 (Figure 2)

BMC/FFM (Women) = 0.0647 (Figure 2)

We first obtain values for the value for the total non-fat density:

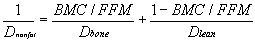D_nonfat (Men) = 1.10914

D_nonfat (Women) = 1.11833

Then,

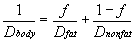
Solving for f, the body fat fraction, and multiplying by 100 for fat percent, gives:

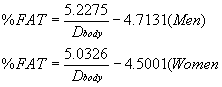
Conclusion

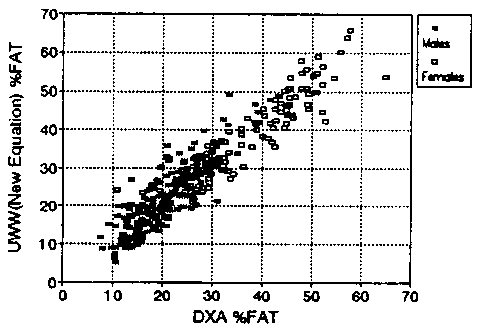
Figure 4 shows the improvement in agreement between UWW and DXA when the new equations are used. Note that the slope of the regression using the new equations is 1.02 compared with 0.84 using the Brozek equation.

Figure 4. Percent body fat by UWW and by DXA compared; new equation set used to calculate UWW result. Regression UWW%FAT = 1.020 * DXA%FAT + 0.68 Data from St Luke's Hospital² and the University of Wisconsin³.

Reference

1. Brozek J, et al. Densitometric analysis of body composition: revision of some quantative assumptions. Ann NY Acad Sci 1963; 110:112-140.
2. Pierson RM, et al. Body Composition Unit, St Luke's Hospital, New York. Private communication.
3. Clark RR, et al. Sports Medicine Centre, Univ of Wisconsin, Madison. Private communication.
4. Nord RH, Payne RK. Body composition by DXA—a review of the technology. Asia Pacific J Clin Nutr 1994;3.
5. Woodard HQ, White DR. The composition of body tissues. Br J Radiology 1986; 59:1209- 1219.
6. White DR, Woodard HQ, Hammond SM. Average soft-tissue and bone models for use in radiation dosimetry. Br J Radiology 1987; 60:907-913.

Copyright © 1996 [Asia Pacific Journal of Clinical Nutrition]. All rights reserved.
Revised: January 19, 1999 .

to the top

0